analytic geometry branch of geometry in which points are represented with respect to a coordinate system, such as Cartesian coordinates , and in which the approach to geometric problems is primarily algebraic. Its most common application is in the representation of equations involving two or three variables as curves in two or three dimensions or surfaces in three dimensions. For example, the linear equation ax + by + c =0 represents a straight line in the xy -plane, and the linear equation ax + by + cz + d =0 represents a plane in space, where a, b, c, and d are constant numbers (coefficients). In this way a geometric problem can be translated into an algebraic problem and the methods of algebra brought to bear on its solution. Conversely, the solution of a problem in algebra, such as finding the roots of an equation or system of equations, can be estimated or sometimes given exactly by geometric means, e.g., plotting curves and surfaces and determining points of intersection.
In plane analytic geometry a line is frequently described in terms of its slope, which expresses its inclination to the coordinate axes; technically, the slope m of a straight line is the (trigonometric) tangent of the angle it makes with the x -axis. If the line is parallel to the x -axis, its slope is zero. Two or more lines with equal slopes are parallel to one another. In general, the slope of the line through the points ( x1 , y1 ) and ( x2 , y2 ) is given by m = ( y2 - y1 ) / ( x2 - x1 ). The conic sections are treated in analytic geometry as the curves corresponding to the general quadratic equation ax2 + bxy + cy2 + dx + ey + f =0, where a, b, … , f are constants and a, b, and c are not all zero.
In solid analytic geometry the orientation of a straight line is given not by one slope but by its direction cosines, λ, μ, and ν, the cosines of the angles the line makes with the x-, y-, and z -axes, respectively; these satisfy the relationship λ 2 +μ 2 +ν 2 = 1. In the same way that the conic sections are studied in two dimensions, the 17 quadric surfaces, e.g., the ellipsoid, paraboloid, and elliptic paraboloid, are studied in solid analytic geometry in terms of the general equation ax2 + by2 + cz2 + dxy + exz + fyz + px + qy + rz + s =0.
The methods of analytic geometry have been generalized to four or more dimensions and have been combined with other branches of geometry. Analytic geometry was introduced by René Descartes in 1637 and was of fundamental importance in the development of the calculus by Sir Isaac Newton and G. W. Leibniz in the late 17th cent. More recently it has served as the basis for the modern development and exploitation of algebraic geometry .
Taken From : http://www.encyclopedia.com/topic/analytic_geometry.aspx#1E1-analytGeo
About Me
Cartesian coordinates
| By Calculus for All
Cartesian coordinates [for René Descartes ], system for representing the relative positions of points in a plane or in space. In a plane, the point P is specified by the pair of numbers ( x,y ) representing the distances of the point from two intersecting straight lines, referred to as the x -axis and the y -axis. The point of intersection of these axes, which are called the coordinate axes, is known as the origin. In rectangular coordinates, the type most often used, the axes are taken to be perpendicular, with the x -axis horizontal and the y -axis vertical, so that the x -coordinate, or abscissa, of P is measured along the horizontal perpendicular from P to the y -axis (i.e., parallel to the x -axis) and the y -coordinate, or ordinate, is measured along the vertical perpendicular from P to the x -axis (parallel to the y -axis). In oblique coordinates the axes are not perpendicular; the abscissa of P is measured along a parallel to the x -axis, and the ordinate is measured along a parallel to the y -axis, but neither of these parallels is perpendicular to the other coordinate axis as in rectangular coordinates. Similarly, a point in space may be specified by the triple of numbers ( x,y,z ) representing the distances from three planes determined by three intersecting straight lines not all in the same plane; i.e., the x -coordinate represents the distance from the yz -plane measured along a parallel to the x -axis, the y -coordinate represents the distance from the xz -plane measured along a parallel to the y -axis, and the z -coordinate represents the distance from the xy -plane measured along a parallel to the z -axis (the axes are usually taken to be mutually perpendicular). Analogous systems may be defined for describing points in abstract spaces of four or more dimensions. Many of the curves studied in classical geometry can be described as the set of points ( x,y ) that satisfy some equation f(x,y) =0. In this way certain questions in geometry can be transformed into questions about numbers and resolved by means of analytic geometry .
Taken From : http://www.encyclopedia.com/topic/Cartesian_coordinates.aspx#1E1-Cartes-coo
Taken From : http://www.encyclopedia.com/topic/Cartesian_coordinates.aspx#1E1-Cartes-coo
Extension of the Trigonometric Functions
| By Calculus for All
The notion of the trigonometric functions can be extended beyond 90° by defining the functions with respect to Cartesian coordinates . Let r be a line of unit length from the origin to the point P ( x,y ), and let θ be the angle r makes with the positive x -axis. The six functions become sin θ = y / r = y, cos θ= x / r = x, tan θ= y / x, cot θ= x / y, sec θ= r / x =1/ x, and csc θ= r / y =1/ y. As θ increases beyond 90°, the point P crosses the y -axis and x becomes negative; in quadrant II the functions are negative except for sin θ and csc θ. Beyond θ=180°, P is in quadrant III, y is also negative, and only tan θ and cot θ are positive, while beyond θ=270° P moves into quadrant IV, x becomes positive again, and cos θ and sec θ are positive. Since the positions of r for angles of 360° or more coincide with those already taken by r as θ increased from 0°, the values of the functions repeat those taken between 0° and 360° for angles greater than 360°, repeating again after 720°, and so on. This repeating, or periodic, nature of the trigonometric functions leads to important applications in the study of such periodic phenomena as light and electricity.
Taken From : http://www.encyclopedia.com/topic/trigonometry.aspx#1E1-trigonom
Taken From : http://www.encyclopedia.com/topic/trigonometry.aspx#1E1-trigonom
The Basic Trigonometric Functions
| By Calculus for All
Trigomometry originated as the study of certain mathematical relations originally defined in terms of the angles and sides of a right triangle, i.e., one containing a right angle (90°). Six basic relations, or trigonometric functions, are defined. If A, B, and C are the measures of the angles of a right triangle ( C =90°) and a, b, and c are the lengths of the respective sides opposite these angles, then the six functions are expressed for one of the acute angles, say A, as various ratios of the opposite side ( a ), the adjacent side ( b ), and the hypotenuse ( c ), as set out in the table. Although the actual lengths of the sides of a right triangle may have any values, the ratios of the lengths will be the same for all similar right triangles, large or small; these ratios depend only on the angles and not on the actual lengths. The functions occur in pairs—sine and cosine, tangent and cotangent, secant and cosecant—called cofunctions. In equations they are usually represented as sin, cos, tan, cot, sec, and csc. Since in ordinary (Euclidean) plane geometry the sum of the angles of a triangle is 180°, angles A and B must add up to 90° and therefore are complementary angles. From the definitions of the functions, it may be seen that sin B =cos A, cos B =sin A, tan B =cot A, and sec B =csc A ; in general, the function of an angle is equal to the cofunction of its complement. Since the hypotenuse ( c ), is always the longest side of a right triangle, the values of the sine and cosine are always between zero and one, the values of the secant and cosecant are always equal to or greater than one, and the values of the tangent and cotangent are unbounded, increasing from zero without limit.
For certain special right triangles the values of the functions may be calculated easily; e.g., in a right triangle whose acute angles are 30° and 60° the sides are in the ratio 1 : 3 : 2, so that sin 30°=cos 60°=1/2, cos 30°=sin 60°= 3 /2, tan 30°=cot 60°=1/ 3 , cot 30°=tan 60°= 3 , sec 30°=csc 60°=2/ 3 , and csc 30°=sec 60°=2. For other angles, the values of the trigonometric functions are usually found from a set of tables or a scientific calculator. For the limiting values of 0° and 90°, the length of one side of the triangle approaches zero while the other approaches that of the hypotenuse, resulting in the values sin 0°=cos 90°=0, cos 0°=sin 90°=1, tan 0°=cot 90°=0, and sec 0°=csc 90°=1; since division by zero is undefined, cot 0°, tan 90°, csc 0°, and sec 90° are all undefined, having infinitely large values.
A general triangle, not necessarily containing a right angle, can also be analyzed by means of trigonometry, and various relationships are found to exist between the sides and angles of the general triangle. For example, in any plane triangle a /sin A = b /sin B = c /sin C. This relationship is known as the Law of Sines. The related Law of Cosines holds that a2 = b2 + c2 -2 bc cos A and the Law of Tangents holds that ( a - b )/( a + b )=[tan 1/2 ( A - B )]/[tan 1/2 ( A + B )]. Each of the trigonometric functions can be represented by an infinite series .
Taken From : http://www.encyclopedia.com/topic/trigonometry.aspx#1E1-trigonom
For certain special right triangles the values of the functions may be calculated easily; e.g., in a right triangle whose acute angles are 30° and 60° the sides are in the ratio 1 : 3 : 2, so that sin 30°=cos 60°=1/2, cos 30°=sin 60°= 3 /2, tan 30°=cot 60°=1/ 3 , cot 30°=tan 60°= 3 , sec 30°=csc 60°=2/ 3 , and csc 30°=sec 60°=2. For other angles, the values of the trigonometric functions are usually found from a set of tables or a scientific calculator. For the limiting values of 0° and 90°, the length of one side of the triangle approaches zero while the other approaches that of the hypotenuse, resulting in the values sin 0°=cos 90°=0, cos 0°=sin 90°=1, tan 0°=cot 90°=0, and sec 0°=csc 90°=1; since division by zero is undefined, cot 0°, tan 90°, csc 0°, and sec 90° are all undefined, having infinitely large values.
A general triangle, not necessarily containing a right angle, can also be analyzed by means of trigonometry, and various relationships are found to exist between the sides and angles of the general triangle. For example, in any plane triangle a /sin A = b /sin B = c /sin C. This relationship is known as the Law of Sines. The related Law of Cosines holds that a2 = b2 + c2 -2 bc cos A and the Law of Tangents holds that ( a - b )/( a + b )=[tan 1/2 ( A - B )]/[tan 1/2 ( A + B )]. Each of the trigonometric functions can be represented by an infinite series .
Taken From : http://www.encyclopedia.com/topic/trigonometry.aspx#1E1-trigonom
The Differential Calculus
| By Calculus for All
The differential calculus arises from the study of the limit of a quotient, Δ y /Δ x, as the denominator Δ x approaches zero, where x and y are variables. y may be expressed as some function of x, or f(x), and Δ y and Δ x represent corresponding increments, or changes, in y and x. The limit of Δ y /Δ x is called the derivative of y with respect to x and is indicated by dy / dx or Dxy : The symbols dy and dx are called differentials (they are single symbols, not products), and the process of finding the derivative of y = f(x) is called differentiation. The derivative dy / dx = df(x) / dx is also denoted by y′, or f′(x). The derivative f′(x) is itself a function of x and may be differentiated, the result being termed the second derivative of y with respect to x and denoted by y″, f″(x), or d2y / dx2 . This process can be continued to yield a third derivative, a fourth derivative, and so on. In practice formulas have been developed for finding the derivatives of all commonly encountered functions. For example, if y = xn , then y′ =nx n - 1 , and if y =sin x, then y′ =cos x (see trigonometry ). In general, the derivative of y with respect to x expresses the rate of change in y for a change in x. In physical applications the independent variable (here x ) is frequently time; e.g., if s = f ( t ) expresses the relationship between distance traveled, s, and time elapsed, t, then s′ = f′ ( t ) represents the rate of change of distance with time, i.e., the speed, or velocity.
Everyday calculations of velocity usually divide the distance traveled by the total time elapsed, yielding the average velocity. The derivative f′ ( t )= ds / dt, however, gives the velocity for any particular value of t, i.e., the instantaneous velocity. Geometrically, the derivative is interpreted as the slope of the line tangent to a curve at a point. If y = f ( x ) is a real-valued function of a real variable, the ratio Δ y /Δ x =( y2 - y1 )/( x2 - x1 ) represents the slope of a straight line through the two points P ( x1 , y1 ) and Q ( x2 , y2 ) on the graph of the function. If P is taken closer to Q, then x1 will approach x2 and Δ x will approach zero. In the limit where Δ x approaches zero, the ratio becomes the derivative dy / dx = f′ ( x ) and represents the slope of a line that touches the curve at the single point Q, i.e., the tangent line. This property of the derivative yields many applications for the calculus, e.g., in the design of optical mirrors and lenses and the determination of projectile paths.
Taken From : http://www.encyclopedia.com/topic/calculus.aspx
Everyday calculations of velocity usually divide the distance traveled by the total time elapsed, yielding the average velocity. The derivative f′ ( t )= ds / dt, however, gives the velocity for any particular value of t, i.e., the instantaneous velocity. Geometrically, the derivative is interpreted as the slope of the line tangent to a curve at a point. If y = f ( x ) is a real-valued function of a real variable, the ratio Δ y /Δ x =( y2 - y1 )/( x2 - x1 ) represents the slope of a straight line through the two points P ( x1 , y1 ) and Q ( x2 , y2 ) on the graph of the function. If P is taken closer to Q, then x1 will approach x2 and Δ x will approach zero. In the limit where Δ x approaches zero, the ratio becomes the derivative dy / dx = f′ ( x ) and represents the slope of a line that touches the curve at the single point Q, i.e., the tangent line. This property of the derivative yields many applications for the calculus, e.g., in the design of optical mirrors and lenses and the determination of projectile paths.
Taken From : http://www.encyclopedia.com/topic/calculus.aspx
The Integral Calculus
| By Calculus for All
The second important kind of limit encountered in the calculus is the limit of a sum of elements when the number of such elements increases without bound while the size of the elements diminishes. For example, consider the problem of determining the area under a given curve y = f ( x ) between two values of x, say a and b. Let the interval between a and b be divided into n subintervals, from a = x0 through x1 , x2 , x3 , … xi - 1 , xi , … , up to xn = b. The width of a given subinterval is equal to the difference between the adjacent values of x, or Δ xi = xi - xi - 1 , where i designates the typical, or i th, subinterval. On each Δ xi a rectangle can be formed of width Δ xi , height yi = f ( xi ) (the value of the function corresponding to the value of x on the right-hand side of the subinterval), and area Δ Ai = f ( xi )Δ xi . In some cases, the rectangle may extend above the curve, while in other cases it may fail to include some of the area under the curve; however, if the areas of all these rectangles are added together, the sum will be an approximation of the area under the curve.
This approximation can be improved by increasing n, the number of subintervals, thus decreasing the widths of the Δ x 's and the amounts by which the Δ A 's exceed or fall short of the actual area under the curve. In the limit where n approaches infinity (and the largest Δ x approaches zero), the sum is equal to the area under the curve: The last expression on the right is called the integral of f ( x ), and f ( x ) itself is called the integrand. This method of finding the limit of a sum can be used to determine the lengths of curves, the areas bounded by curves, and the volumes of solids bounded by curved surfaces, and to solve other similar problems.
An entirely different consideration of the problem of finding the area under a curve leads to a means of evaluating the integral. It can be shown that if F ( x ) is a function whose derivative is f ( x ), then the area under the graph of y = f ( x ) between a and b is equal to F ( b ) - F ( a ). This connection between the integral and the derivative is known as the Fundamental Theorem of the Calculus. Stated in symbols: The function F ( x ), which is equal to the integral of f ( x ), is sometimes called an antiderivative of f ( x ), while the process of finding F ( x ) from f ( x ) is called integration or antidifferentiation. The branch of calculus concerned with both the integral as the limit of a sum and the integral as the antiderivative of a function is known as the integral calculus. The type of integral just discussed, in which the limits of integration, a and b, are specified, is called a definite integral. If no limits are specified, the expression is an indefinite integral. In such a case, the function F ( x ) resulting from integration is determined only to within the addition of an arbitrary constant C, since in computing the derivative any constant terms having derivatives equal to zero are lost; the expression for the indefinite integral of f ( x ) is The value of the constant C must be determined from various boundary conditions surrounding the particular problem in which the integral occurs. The calculus has been developed to treat not only functions of a single variable, e.g., x or t, but also functions of several variables. For example, if z = f ( x,y ) is a function of two independent variables, x and y, then two different derivatives can be determined, one with respect to each of the independent variables. These are denoted by ∂ z /∂ x and ∂ z /∂ y or by Dxz and Dyz. Three different second derivatives are possible, ∂ 2z /∂ x2 , ∂ 2z /∂ y2 , and ∂ 2z /∂ x ∂ y =∂ 2z /∂ y ∂ x. Such derivatives are called partial derivatives. In any partial differentiation all independent variables other than the one being considered are treated as constants.
Taken From : http://www.encyclopedia.com/topic/calculus.aspx
This approximation can be improved by increasing n, the number of subintervals, thus decreasing the widths of the Δ x 's and the amounts by which the Δ A 's exceed or fall short of the actual area under the curve. In the limit where n approaches infinity (and the largest Δ x approaches zero), the sum is equal to the area under the curve: The last expression on the right is called the integral of f ( x ), and f ( x ) itself is called the integrand. This method of finding the limit of a sum can be used to determine the lengths of curves, the areas bounded by curves, and the volumes of solids bounded by curved surfaces, and to solve other similar problems.
An entirely different consideration of the problem of finding the area under a curve leads to a means of evaluating the integral. It can be shown that if F ( x ) is a function whose derivative is f ( x ), then the area under the graph of y = f ( x ) between a and b is equal to F ( b ) - F ( a ). This connection between the integral and the derivative is known as the Fundamental Theorem of the Calculus. Stated in symbols: The function F ( x ), which is equal to the integral of f ( x ), is sometimes called an antiderivative of f ( x ), while the process of finding F ( x ) from f ( x ) is called integration or antidifferentiation. The branch of calculus concerned with both the integral as the limit of a sum and the integral as the antiderivative of a function is known as the integral calculus. The type of integral just discussed, in which the limits of integration, a and b, are specified, is called a definite integral. If no limits are specified, the expression is an indefinite integral. In such a case, the function F ( x ) resulting from integration is determined only to within the addition of an arbitrary constant C, since in computing the derivative any constant terms having derivatives equal to zero are lost; the expression for the indefinite integral of f ( x ) is The value of the constant C must be determined from various boundary conditions surrounding the particular problem in which the integral occurs. The calculus has been developed to treat not only functions of a single variable, e.g., x or t, but also functions of several variables. For example, if z = f ( x,y ) is a function of two independent variables, x and y, then two different derivatives can be determined, one with respect to each of the independent variables. These are denoted by ∂ z /∂ x and ∂ z /∂ y or by Dxz and Dyz. Three different second derivatives are possible, ∂ 2z /∂ x2 , ∂ 2z /∂ y2 , and ∂ 2z /∂ x ∂ y =∂ 2z /∂ y ∂ x. Such derivatives are called partial derivatives. In any partial differentiation all independent variables other than the one being considered are treated as constants.
Taken From : http://www.encyclopedia.com/topic/calculus.aspx
Finsler geometry
Wednesday, April 21, 2010 | By Calculus for All
Finsler geometry has the Finsler manifold as the main object of study — this is a differential manifold with a Finsler metric, i.e. a Banach norm defined on each tangent space. A Finsler metric is a much more general structure than a Riemannian metric. A Finsler structure on a manifold M is a function F : TM → [0,∞) such that:
1. F(x, my) = |m|F(x,y) for all x, y in TM,
2. F is infinitely differentiable in TM − {0},
3. The vertical Hessian of F2 is positive definite.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
1. F(x, my) = |m|F(x,y) for all x, y in TM,
2. F is infinitely differentiable in TM − {0},
3. The vertical Hessian of F2 is positive definite.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
Riemannian geometry
| By Calculus for All
Riemannian geometry studies Riemannian manifolds, smooth manifolds with a Riemannian metric, a notion of a distance expressed by means of a smooth positive definite symmetri bilinear form defined on the tangent space at each point. Riemannian geometry generalizes Euclidean geometry to spaces that are not necessarily flat, although they still resemble the Euclidean space at each point "infinitesimally", i.e. in the first order of approximation. Various concepts based on length, such as the arc length of curves, area of plane regions, and volume of solids all admit natural analogues in Riemannian geometry. The notion of a directional derivative of a function from multivariable calculus is extended in Riemannian geometry to the notion of a covariant derivative of a tensor. Many concepts and techniques of analysis and differential equations have been generalized to the setting of Riemannian manifolds.
A distance-preserving diffeomorphism between Riemannian manifolds is called an isometry. This notion can also be defined locally, i.e. for small neighborhoods of points. Any two regular curves are locally isometric. However, Theorema Egregium of Gauss showed that already for surfaces, the existence of a local isometry imposes strong compatibility conditions on their metrics: the Gaussian curvatures at the corresponding points must be the same. In higher dimensions, the Riemann curvature tensor is an important pointwise invariant associated to a Riemannian manifold that measures how close it is to being flat. An important class of Riemannian manifolds is formed by the Riemannian symmetric spaces, whose curvature is not necessarily constant. They are the closest to the "ordinary" plane and space considered in Euclidean and non-Euclidean geometry.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
A distance-preserving diffeomorphism between Riemannian manifolds is called an isometry. This notion can also be defined locally, i.e. for small neighborhoods of points. Any two regular curves are locally isometric. However, Theorema Egregium of Gauss showed that already for surfaces, the existence of a local isometry imposes strong compatibility conditions on their metrics: the Gaussian curvatures at the corresponding points must be the same. In higher dimensions, the Riemann curvature tensor is an important pointwise invariant associated to a Riemannian manifold that measures how close it is to being flat. An important class of Riemannian manifolds is formed by the Riemannian symmetric spaces, whose curvature is not necessarily constant. They are the closest to the "ordinary" plane and space considered in Euclidean and non-Euclidean geometry.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
Symplectic geometry
| By Calculus for All
Symplectic geometry is the study of symplectic manifolds. An almost symplectic manifold is a differentiable manifold equipped with a smoothly varying non-degenerate skew-symmetric bilinear form on each tangent space, i.e., a nondegenerate 2-form ω, called the symplectic form. A symplectic manifold is an almost symplectic manifold for which the symplectic form ω is closed: dω = 0.
A diffeomorphism between two symplectic manifolds which preserves the symplectic form is called a symplectomorphism. Non-degenerate skew-symmetric bilinear forms can only exist on even dimensional vector spaces, so symplectic manifolds necessarily have even dimension. In dimension 2, a symplectic manifold is just a surface endowed with an area form and a symplectomorphism is an area-preserving diffeomorphism. The phase space of a mechanical system is a symplectic manifold and they made an implicit appearance already in the work of Lagrange on analytical mechanics and later in Jacobi's and Hamilton's formulation of classical mechanics.
By contrast with Riemannian geometry, where the curvature provides a local invariant of Riemannian manifolds, Darboux's theorem states that all symplectic manifolds are locally isomorphic. The only invariants of a symplectic manifold are global in nature and topological aspects play a prominent role in symplectic geometry. The first result in symplectic topology is probably the Poincaré-Birkhoff theorem, conjectured by Henri Poincaré and proved by George Birkhoff in 1912. It claims that if an area preserving map of an annulus twists each boundary component in opposite directions, then the map has at least two fixed points.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
A diffeomorphism between two symplectic manifolds which preserves the symplectic form is called a symplectomorphism. Non-degenerate skew-symmetric bilinear forms can only exist on even dimensional vector spaces, so symplectic manifolds necessarily have even dimension. In dimension 2, a symplectic manifold is just a surface endowed with an area form and a symplectomorphism is an area-preserving diffeomorphism. The phase space of a mechanical system is a symplectic manifold and they made an implicit appearance already in the work of Lagrange on analytical mechanics and later in Jacobi's and Hamilton's formulation of classical mechanics.
By contrast with Riemannian geometry, where the curvature provides a local invariant of Riemannian manifolds, Darboux's theorem states that all symplectic manifolds are locally isomorphic. The only invariants of a symplectic manifold are global in nature and topological aspects play a prominent role in symplectic geometry. The first result in symplectic topology is probably the Poincaré-Birkhoff theorem, conjectured by Henri Poincaré and proved by George Birkhoff in 1912. It claims that if an area preserving map of an annulus twists each boundary component in opposite directions, then the map has at least two fixed points.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
Complex and Kähler geometry
| By Calculus for All
Complex differential geometry is the study of complex manifolds. An almost complex manifold is a real manifold M, endowed with a tensor of type (1,1), i.e. a vector bundle endomorphism (called an almost complex structure)
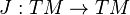 , such that J2 = − 1.
, such that J2 = − 1.
It follows from this definition that an almost complex manifold is even dimensional.
An almost complex manifold is called complex if NJ = 0, where NJ is a tensor of type (2,1) related to J, called the Nijenhuis tensor (or sometimes the torsion). An almost complex manifold is complex if and only if it admits a holomorphic coordinate atlas. An almost Hermitian structure is given by an almost complex structure J, along with a riemannian metric g, satisfying the compatibility condition g(JX,JY) = g(X,Y). An almost hermitian structure defines naturally a differential 2-form ωJ,g(X,Y): = g(JX,Y). The following two conditions are equivalent:
1. NJ = 0 and dω = 0,
2.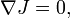
where is the Levi-Civita connection of g. In this case, (J,g) is called a Kähler structure, and a Kähler manifold is a manifold endowed with a Kähler structure. In particular, a Kähler manifold is both a complex and a symplectic manifold. A large class of Kähler manifolds (the class of Hodge manifolds) is given by all the smooth complex projective varieties.
is the Levi-Civita connection of g. In this case, (J,g) is called a Kähler structure, and a Kähler manifold is a manifold endowed with a Kähler structure. In particular, a Kähler manifold is both a complex and a symplectic manifold. A large class of Kähler manifolds (the class of Hodge manifolds) is given by all the smooth complex projective varieties.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
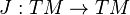 , such that J2 = − 1.
, such that J2 = − 1.It follows from this definition that an almost complex manifold is even dimensional.
An almost complex manifold is called complex if NJ = 0, where NJ is a tensor of type (2,1) related to J, called the Nijenhuis tensor (or sometimes the torsion). An almost complex manifold is complex if and only if it admits a holomorphic coordinate atlas. An almost Hermitian structure is given by an almost complex structure J, along with a riemannian metric g, satisfying the compatibility condition g(JX,JY) = g(X,Y). An almost hermitian structure defines naturally a differential 2-form ωJ,g(X,Y): = g(JX,Y). The following two conditions are equivalent:
1. NJ = 0 and dω = 0,
2.
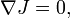
where
 is the Levi-Civita connection of g. In this case, (J,g) is called a Kähler structure, and a Kähler manifold is a manifold endowed with a Kähler structure. In particular, a Kähler manifold is both a complex and a symplectic manifold. A large class of Kähler manifolds (the class of Hodge manifolds) is given by all the smooth complex projective varieties.
is the Levi-Civita connection of g. In this case, (J,g) is called a Kähler structure, and a Kähler manifold is a manifold endowed with a Kähler structure. In particular, a Kähler manifold is both a complex and a symplectic manifold. A large class of Kähler manifolds (the class of Hodge manifolds) is given by all the smooth complex projective varieties.Taken From http://en.wikipedia.org/wiki/Differential_geometry
Contact geometry
| By Calculus for All
Contact geometry deals with certain manifolds of odd dimension. It is close to symplectic geometry and like the latter, it originated in questions of classical mechanics. A contact structure on a (2n+1)-dimensional manifold M is given by a smooth hyperplane field H in the tangent bundle that is as far as possible from being associated with the level sets of a differentiable function on M (the technical term is "completely nonintegrable tangent hyperplane distribution"). Near each point p, a hyperplane distribution is determined by a nowhere vanishing 1-form α, which is unique up to multiplication by a nowhere vanishing function:
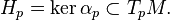
A local 1-form on M is a contact form if the restriction of its exterior derivative to H is a non-degenerate 2-form and thus induces a symplectic structure on Hp at each point. If the distribution H can be defined by a global 1-form α then this form is contact if and only if the top-dimensional form
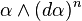
is a volume form on M, i.e. does not vanish anywhere. A contact analogue of the Darboux theorem holds: all contact structures on an odd-dimensional manifold are locally isomorphic and can be brought to a certain local normal form by a suitable choice of the coordinate system.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
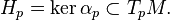
A local 1-form on M is a contact form if the restriction of its exterior derivative to H is a non-degenerate 2-form and thus induces a symplectic structure on Hp at each point. If the distribution H can be defined by a global 1-form α then this form is contact if and only if the top-dimensional form
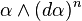
is a volume form on M, i.e. does not vanish anywhere. A contact analogue of the Darboux theorem holds: all contact structures on an odd-dimensional manifold are locally isomorphic and can be brought to a certain local normal form by a suitable choice of the coordinate system.
Taken From http://en.wikipedia.org/wiki/Differential_geometry
Taylor polynomials and Taylor series
| By Calculus for All
The derivative gives the best possible linear approximation, but this can be very different from the original function. One way of improving the approximation is to take a quadratic approximation. That is to say, the linearization of a real-valued function f(x) at the point x0 is a linear polynomial a + b(x - x0), and it may be possible to get a better approximation by considering a quadratic polynomial a + b(x - x0) + c(x - x0)². Still better might be a cubic polynomial a + b(x - x0) + c(x - x0)² + d(x - x0)³, and this idea can be extended to arbitrarily high degree polynomials. For each one of these polynomials, there should be a best possible choice of coefficients a, b, c, and d that makes the approximation as good as possible.
For a, the best possible choice is always f(x0), and for b, the best possible choice is always f'(x0). For c, d, and higher-degree coefficients, these coefficients are determined by higher derivatives of f. c should always be f''(x0)/2, and d should always be f'''(x0)/3!. Using these coefficients gives the Taylor polynomial of f. The Taylor polynomial of degree d is the polynomial of degree d which best approximates f, and its coefficients can be found by a generalization of the above formulas. Taylor’s theorem gives a precise bound on how good the approximation is. If f is a polynomial of degree less than or equal to d, then the Taylor polynomial of degree d equals f.
The limit of the Taylor polynomials is an infinite series called the Taylor series. The Taylor series is frequently a very good approximation to the original function. Functions which are equal to their Taylor series are called analytic functions . It is impossible for functions with discontinuities or sharp corners to be analytic, but there are smooth functions which are not analytic.
Taken From http://en.wikipedia.org/wiki/Differential_calculus#Calculus_of_variations
For a, the best possible choice is always f(x0), and for b, the best possible choice is always f'(x0). For c, d, and higher-degree coefficients, these coefficients are determined by higher derivatives of f. c should always be f''(x0)/2, and d should always be f'''(x0)/3!. Using these coefficients gives the Taylor polynomial of f. The Taylor polynomial of degree d is the polynomial of degree d which best approximates f, and its coefficients can be found by a generalization of the above formulas. Taylor’s theorem gives a precise bound on how good the approximation is. If f is a polynomial of degree less than or equal to d, then the Taylor polynomial of degree d equals f.
The limit of the Taylor polynomials is an infinite series called the Taylor series. The Taylor series is frequently a very good approximation to the original function. Functions which are equal to their Taylor series are called analytic functions . It is impossible for functions with discontinuities or sharp corners to be analytic, but there are smooth functions which are not analytic.
Taken From http://en.wikipedia.org/wiki/Differential_calculus#Calculus_of_variations
Mean value theorem
| By Calculus for All
The mean value theorem gives a relationship between values of the derivative and values of the original function. If f(x) is a real-valued function and a and b are numbers with a < b, then the mean value theorem says that under mild hypotheses, the slope between the two points (a, f(a)) and (b, f(b)) is equal to the slope of the tangent line to f at some point c between a and b. In other words,

In practice, what the mean value theorem does is control a function in terms of its derivative. For instance, suppose that f has derivative equal to zero at each point. This means that its tangent line is horizontal at every point, so the function should also be horizontal. The mean value theorem proves that this must be true: The slope between any two points on the graph of f must equal the slope of one of the tangent lines of f. All of those slopes are zero, so any line from one point on the graph to another point will also have slope zero. But that says that the function does not move up or down, so it must be a horizontal line. More complicated conditions on the derivative lead to less precise but still highly useful information about the original function.
Taken From http://en.wikipedia.org/wiki/Differential_calculus#Calculus_of_variations

In practice, what the mean value theorem does is control a function in terms of its derivative. For instance, suppose that f has derivative equal to zero at each point. This means that its tangent line is horizontal at every point, so the function should also be horizontal. The mean value theorem proves that this must be true: The slope between any two points on the graph of f must equal the slope of one of the tangent lines of f. All of those slopes are zero, so any line from one point on the graph to another point will also have slope zero. But that says that the function does not move up or down, so it must be a horizontal line. More complicated conditions on the derivative lead to less precise but still highly useful information about the original function.
Taken From http://en.wikipedia.org/wiki/Differential_calculus#Calculus_of_variations
Physics
| By Calculus for All
Physics
Calculus is of vital importance in physics: many physical processes are described by equations involving derivatives, called differential equations . Physics is particularly concerned with the way quantities change and evolve over time, and the concept of the "time derivative" — the rate of change over time — is essential for the precise definition of several important concepts. In particular, the time derivatives of an object's position are significant in Newtonian physics:
• Velocity is the derivative (with respect to time) of an object's displacement (distance from the original position)
• Acceleration is the derivative (with respect to time) of an object's velocity, that is, the second derivative (with respect to time) of an object's position.
For example, if an object's position on a line is given by

then the object's velocity is
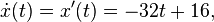
and the object's acceleration is

which is constant.
Taken From http://en.wikipedia.org/wiki/Differential_calculus#Calculus_of_variations
Calculus is of vital importance in physics: many physical processes are described by equations involving derivatives, called differential equations . Physics is particularly concerned with the way quantities change and evolve over time, and the concept of the "time derivative" — the rate of change over time — is essential for the precise definition of several important concepts. In particular, the time derivatives of an object's position are significant in Newtonian physics:
• Velocity is the derivative (with respect to time) of an object's displacement (distance from the original position)
• Acceleration is the derivative (with respect to time) of an object's velocity, that is, the second derivative (with respect to time) of an object's position.
For example, if an object's position on a line is given by

then the object's velocity is
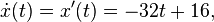
and the object's acceleration is

which is constant.
Taken From http://en.wikipedia.org/wiki/Differential_calculus#Calculus_of_variations
Differential equations
| By Calculus for All
Main article: Differential Equation
A differential equation is relation between a collection of functions and their derivatives. An ordinary differential equation is a differential equation that relates functions of one variable to their derivatives with respect to that variable. A partial differential equation is a differential equation that relates functions of more than one variable to their partial derivatives. Differential equations arise naturally in the physical sciences, in mathematical modelling, and within mathematics itself. For example, Newton Seconds Law , which describes the relationship between acceleration and position, can be stated as the ordinary differential equation
The heat equation in one space variable, which describes how heat diffuses through a straight rod, is the partial differential equation

Here u(x, t) is the temperature of the rod at position x and time t and α is a constant that depends on how fast heat diffuses through the rod.
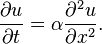
Taken From http://en.wikipedia.org/wiki/Differential_calculus
A differential equation is relation between a collection of functions and their derivatives. An ordinary differential equation is a differential equation that relates functions of one variable to their derivatives with respect to that variable. A partial differential equation is a differential equation that relates functions of more than one variable to their partial derivatives. Differential equations arise naturally in the physical sciences, in mathematical modelling, and within mathematics itself. For example, Newton Seconds Law , which describes the relationship between acceleration and position, can be stated as the ordinary differential equation
The heat equation in one space variable, which describes how heat diffuses through a straight rod, is the partial differential equation

Here u(x, t) is the temperature of the rod at position x and time t and α is a constant that depends on how fast heat diffuses through the rod.
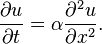
Taken From http://en.wikipedia.org/wiki/Differential_calculus
The derivative
| By Calculus for All

The derivative
Main article: Derivative
Suppose that x and y are real numbers and that y is a function of x, that is, for every value of x, we can determine the value of y. This relationship is written as: y = f(x). Where f(x) is the equation for a straight line, y = m x + b, where m and b are real numbers that determine the locus of the line in Cartesian coordinates. m is called the slope and is given by:

where the symbol Δ (the uppercase form of the Greek letter Delta) is an abbreviation for "change in". It follows that Δy = m Δx.
In linear functions the derivative of f at the point x is the best possible approximation to the idea of the slope of f at the point x. It is usually denoted f'(x) or dy/dx. Together with the value of f at x, the derivative of f determines the best linear approximation, or linearization, of f near the point x. This latter property is usually taken as the definition of the derivative. Derivatives cannot be calculated in nonlinear functions because they do not have a well-defined slope.
A closely related notion is the differential of a function.
The tangent line at (x, f(x))
When x and y are real variables, the derivative of f at x is the slope of the tangent line to the graph of f' at x. Because the source and target of f are one-dimensional, the derivative of f is a real number. If x and y are vectors, then the best linear approximation to the graph of f depends on how f changes in several directions at once. Taking the best linear approximation in a single direction determines a partial derivative, which is usually denoted ∂y/∂x. The linearization of f in all directions at once is called the total derivative. It is a linear transformation, and it determines the hyperplane that most closely approximates the graph of f. This hyperplane is called the oscilating hyperplane; it is conceptually the same idea as taking tangent lines in all directions at once.
taken from http://en.wikipedia.org/wiki/Differential_calculus
2010 - Time for Calculus is proudly powered by Blogger
Blogger Template created by Anshul
Design By Templatelite.com
Blogger Template created by Anshul
Design By Templatelite.com